So, recall from the great circle explanation that
when the globe isn't spinning, something shot East or West will curve away
onto a path that goes around the center of the sphere. Now we set the sphere
spinning and see what happens to attempts to fire along a great circle.

|
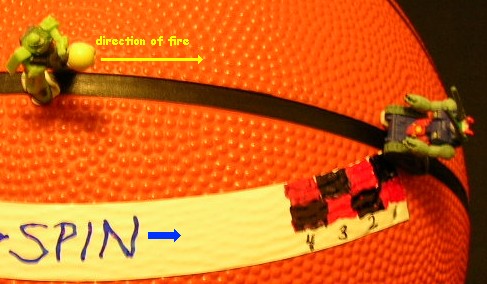
Our two opponents from the North/South example have
repositioned themselves on the bumpy orange planet. At the moment, the blue
and gray mech is farther North than the green mech, and is shooting due West
in order to get a great circle path shown in black. As in the case of the
North/South combat, the only real force on the projectile is directed towards
the center of the planet. In this particular case, the latitude of the green
mech is spinning 4 units faster than the latitude of the blue and gray
mech. We are going to assume that the shot leaves the barrel going faster
than the rotation speed of the shooter, so it really is traveling to the west
from the point of view of someone floating nearby.
Important Note: Remember, because gravity is pulling the shot towards
the center of the planet, the shooter is expecting it to curve to the left as
it follows a great circle. He's aiming due West to hit a target to the
Southwest.
|

|
Because of the faster spin at the white latitude, the green mech moves 4
units farther East of where a simple great circle shot would have landed, and
the projectile seems to have curved off to the right...or rather, it hasn't
curved as far to the left as would be expected if the only force were
gravity. That's the key to the weirdness here, we don't just have the frame
effect in action, it's mixing up with a real center-directed force, and
making it seem to observers on the ground that there's an extra force that
partially cancels the center-directed force.
Of course, as shown in the two dimensional case, speed
matters. At lower speeds, a shot to the west might miss to the left instead,
or even hit right on target if just the right speed was picked.
|
|
The green mech chases the blue and gray mech along the great circle and
prepares to return fire. But he's a slow learner, and just fires due East,
to get a great circle path onto where his foe now sits. Since he's firing in
the direction of spin, the speed doesn't matter as much since the deflection
will always be to the right, but it still matters in terms of figuring out
exactly how much deflection there is.
|

|
Because the ground at the white latitude is moving 4 units faster than the
ground where the green mech stands, his target is 4 units East of where the
projectile goes. As before, the great circle itself has moved to the East.
So, again, it looks like some mysterious force is pushing his shots to the
right. In this case, it's adding to the great circle curvature.
|
If the battle were to move to the Southern Hemisphere, firing West at a high
enough speed would still make the shots miss to the West, but now it would
look like a deflection to the left. Same thing with shooting East, it would
bend left to land West of the target.
Note that if things are moving short distances so that the latitude doesn't
change significantly, you can think of this more in terms of the Two Dimensional case. Something shot East will deflect
outward from the axis, which translates into "right and up" (with the "up"
part interacting with gravity). Something shot slowly to the West will also
seem to deflect outward from the axis, or "left and up", while something shot
quickly enough to the West will deflect inward towards the axis, or "right
and down". Keep in mind that the ground drops away faster than the downward
part of the frame effect can kick in (it's a "size of the planet" scale
effect, not a "size of the baseball diamond" scale thing), so it's not like
shooting something slowly to the west will make it nosedive.
Back to the Main Coriolis force Page.
|